Difference between revisions of "Effect Modification"
Bosmana fem (talk | contribs) m (→The concept Explained) |
Bosmana fem (talk | contribs) m |
||
| (One intermediate revision by the same user not shown) | |||
| Line 95: | Line 95: | ||
=References= | =References= | ||
<references/> | <references/> | ||
| + | |||
| + | |||
| + | =Credits= | ||
| + | ===FEM Editor 2007=== | ||
| + | * Naomi Boxall | ||
| + | ===Original Author=== | ||
| + | * Alain Moren | ||
| + | ===FEM Contributors=== | ||
| + | * Arnold Bosman | ||
| + | * Sabrina Bacci | ||
| + | * Lisa Lazareck | ||
| + | * Naomi Boxall | ||
| + | |||
| + | [[Category:Effect Modification and Confounding]] | ||
Latest revision as of 21:27, 22 March 2023
Contents
Effect Modification Explained
If the magnitude of the risk ratio, rate ratio, odds ratio or risk difference) varies in different subgroups (strata) of the study population, there is effect modification. This differs from confounding, where we generally believe that the measure of effect (i.e. the RR, OR etc.) will be the same in each of the strata defined by levels of the confounding variable. Where the measure of effect does differ by the effect modifying variable, it is unreasonable to combine the results from the different strata (as is the case using the Mantel-Haenzel methods for a confounding variable).
Cohort study (hypothetical)
In the hypothetical cohort study carried out to measure the effectiveness of a vaccine on preventing occurrence of disease X; vaccine effectiveness (VE) can be derived from the risk ratio (RR) using the formula: VE = (1 - RR) *100 (to express VE as a percentage).
| Vaccination | Denominator | Cases | Risk/1000 | Risk Ratio | VE (%) |
|---|---|---|---|---|---|
| Yes | 301545 | 150 | 0.50 | 0.27 | 71 |
| No | 298655 | 515 | 1.72 |
The risk ratio for the entire cohort of 0.29 implies a VE of 71%. But we also looked at VE across different age groups of the population. Within each age group, a risk ratio comparing the risk of disease X between the vaccinated and unvaccinated was computed.
| Age Group | Vaccination | Denominator | Cases | Risk/1000 | Risk Ratio | VE (%) |
|---|---|---|---|---|---|---|
| < 1 year | Yes | 35625 | 38 | 1.07 | 0.87 | 13 |
| No | 24375 | 30 | 1.23 | |||
| 1 - 4 years | Yes | 44220 | 34 | 0.77 | 0.42 | 58 |
| No | 46780 | 86 | 1.84 | |||
| 5 - 9 years | Yes | 78200 | 50 | 0.64 | 0.19 | 81 |
| No | 75000 | 250 | 3.33 | |||
| 10 - 24 years | Yes | 83400 | 18 | 0.22 | 0.15 | 85 |
| No | 82600 | 120 | 1.45 | |||
| > 24 years | Yes | 60100 | 10 | 0.17 | 0.40 | 60 |
| No | 69900 | 29 | 0.41 |
We observe that the risk ratio ranges from 0.15 to 0.87 according to various age groups, consequently, neither is VE equal for the various age groups. This suggests that age is modifying the protective effect of the vaccine. Age is called an effect modifier.
Since the data suggest different vaccine effectiveness by age group it would not be logical to summarise the table and give only an overall vaccine effectiveness (e.g. 71%). It is important to describe the VE by age groups. When effect modification is suggested by the data, it is important to present stratum specific results that provide more information than an overall effect.
Case control study
The same reasoning can be applied to a case-control study. A case-control study conducted in France in 1995 suggest that storing eggs for longer than 2 weeks in the home increases the risk of gastroenteritis (OR = 3.8) in children [1]. However if the analysis is stratified in two seasons, summer and others, the odds ratio is higher in summer (OR = 6) than in other seasons (OR = 2.3), suggesting that the increased risk of gastroenteritis with duration of home eggs storage expresses itself differently according to the season. Here, season is an effect modifier of the association between duration of storage of eggs in the home and the occurrence of Salmonella enteritidis gastroenteritis.
| Cases | Controls | OR | 95% CI | ||
|---|---|---|---|---|---|
| Duration of storage | |||||
| Overall | ≥ 2 weeks | 12 | 2 | 6 | 1.3 - 26.8 |
| < 2 weeks | 52 | 64 | |||
| Summer | ≥ 2 weeks | 19 | 5 | 3.8 | 1.4 - 10.2 |
| < 2 weeks | 84 | 100 | |||
| Seasons other than summer | ≥ 2 weeks | 7 | 3 | 2.3 | 0.6 - 9.0 |
| < 2 weeks | 32 | 36 |
Specific statistical methods are used to look for effect modification and test the homogeneity of stratum-specific risk ratios or odds ratios. The most popular tests include the Woolf test, Breslow-Day, Χ2 for trends, etc. Details of the various methods can be found in referenced books and articles [2].
Assessing risk differences between exposed and unexposed cohorts
In the two above examples, effect modification was assessed by comparing the risk ratios or odds ratios between different sub-groups (strata) of a population. However we sometimes use risk difference to identify how risk varies between exposed and unexposed cohorts.
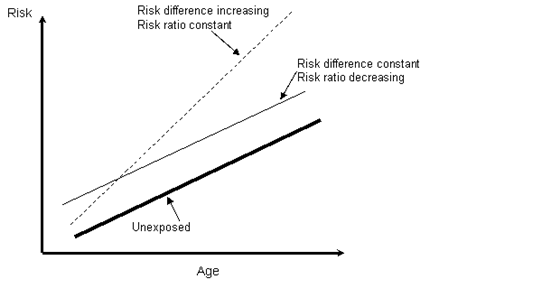
The following example is a classic illustration of the difficulty to conclude on the presence or not of effect modification according to the type of effect measure we use (risk ratio or risk difference). In the figure, the risk of hypothetical disease X is compared between exposed and unexposed according to age. The risk increases with age linearly among unexposed (bold line). For the exposed groups two alternatives are presented. First the line representing the increase of risk with age among exposed (plain line) is parallel to that of unexposed. The risk difference (RD) is constant and the RR decreases with age. Alternatively (doted line) if risk increases with a bigger slope among exposed, RD increases with age and RR is constant. This is why some authors would use the term effect-measure modification rather than effect modification to make sure that the type of effect measure (RR or RD) is specified [3], [4]. Some also refer to "an effect modifier of the risk difference" or alternatively of the risk ratio.
Example (hypothetical)
A cohort study collects information on drinking, exposure to ceramic dust and subsequent liver cancer. The table shows the risk (over 1 year, per 100,000 persons) derived from the study.
| No ceramic dust | Ceramic dust | |
|---|---|---|
| Drinker | 10 | 50 |
| Non-drinker | 1 | 5 |
Among those exposed to ceramic dust, the relative risk of liver cancer between drinkers and non-drinkers is 10 (50/5). Among the unexposed, the relative risk between drinkers and non-drinkers is 10 (10/1).
The risk difference between the drinkers and non-drinkers who are not exposed to ceramic dust is 10-1=9/100,000 persons. The risk difference between drinkers and non-drinkers who are exposed to ceramic dust is 50-5=45/100,000 persons.
The difference in effect modification between these scales reflects statistical interaction - which refers to the deviation from the underlying model. This is different from biological interaction.
References
- ↑ Delarocque-Astagneau, E., Desenclos JC, Bouvet P, Grimont PA. Risk factors for the occurrence of sporadic Salmonella enterica serotype enteritidis infections in children in France: a national case-control study. Epidemiol Infect 1998 Dec;121(3):561-7.
- ↑ B.R.Kirkwood, J.A.C.Sterne. Medical Statistics. Second ed. Massachusetts: Blackwell Science Ltd; 2003.
- ↑ A Dictionary of Epidemiology. Fifth ed. New York: Oxford University Press; 2008.
- ↑ K.J.Rothman, S.Greenland, T.L.Lash. Modern Epidemiology. Third ed. Philadelphia, USA: Lipincott Williams and Wilkins; 2008.
Credits
FEM Editor 2007
- Naomi Boxall
Original Author
- Alain Moren
FEM Contributors
- Arnold Bosman
- Sabrina Bacci
- Lisa Lazareck
- Naomi Boxall
Root > Assessing the burden of disease and risk assessment > Field Epidemiology > Measurement in Field Epidemiology > Problems with Measurement > Bias > Effect Modification and Confounding